THE SOURCE OF SCIENTIFIC KNOWLEDGE ( ILM AL_LADUN)

"We had taught him Knowledge from Our side (ilm al-ladun)."

—God (18:65)

"Now the discoveries of modern science are all Hidden Knowledge, that’s how you’re going to see it."

—Master Ahmet Kayhan (TPM p. 255.)

God-given Knowledge
The present article is an attempt to clarify the Master's saying in the epigraph. "Knowledge from Our side" (ilm al-ladun) is usually taken to mean secret knowledge. Master Kayhan, however, regarded all newly obtained knowledge—whether physical or spiritual—as (previously) hidden knowledge. Both were of value to him. The operative Verse here is: "Previously, you didn't know this" (12:3)—i.e., now you have been apprised of it. Hence, Sufic knowledge is more comprehensive than physical knowledge, which it includes and transcends.  
"Knowledge from God's side" means that knowledge—true knowledge—is imparted, granted, or "given," by God. God opens a window, as it were, granting access to the relevant part of His all-encompassing Knowledge/Science (ilm).
The act of scientific creativity is what drives science, and yet we still know little of its nature. One thing is certain: there is something revelatory about it. We sometimes tend to forget that the chemist Kekulé discovered the benzene ring, and physicist Niels Bohr his model of the atom, in dreams. (The first saw a snake swallowing its tail, the latter a planetary system.) In a letter to psychologist Carl G. Jung, physicist Wolfgang Pauli expressed his own experience with dreams: "Later, however, I came to recognize the objective nature of these dreams or fantasies."

“The discoveries of modern science are all

‘Hidden Knowledge/Knowledge from God's side’

(ilm al-ladun)”

Let us first look at some examples. For those not well-acquainted with science, these are all famous scientists.

Examples: "Eureka!" Moments
"Eureka," of course, refers to Archimedes, the scientist of Antiquity, who bolted from a bath-house naked upon discovering the principle of buoyancy.
Henri Poincaré (mathematics)
Poincaré has left us perhaps the most detailed account of how a mathematician's mind works. Here he is talking about his work on a certain class of mathematical functions:

"For fifteen days I strove to prove that there could not be any functions like those I have since called Fuchsian functions. I was then very ignorant; every day I seated myself at my work table, stayed an hour or two, tried a great number of combinations and reached no results. ...

Just at this time I left Caen, where I was then living, to go on a geologic excursion under the auspices of the school of mines. The changes of travel made me forget my mathematical work. Having reached Coutances, we entered an omnibus to go some place or other. At the moment when I put my foot on the step the idea [resolving the matter] came to me, without anything in my former thoughts seeming to have paved the way for it... I did not verify the idea; I should not have had time, as, upon taking my seat in the omnibus, I went on with a conversation already commenced, but I felt a perfect certainty. ...

Then I turned my attention to the study of some arithmetical questions apparently without much success and without a suspicion of any connection with my preceding researches. Disgusted with my failure, I went to spend a few days at the seaside, and thought of something else. One morning, walking on the bluff, the idea came to me, with just the same characteristics of brevity, suddenness and immediate certainty, that [resolved the issue].

Returned to Caen, I meditated on this result and deduced the consequences... Naturally I set myself to form all these functions. I made a systematic attack upon them and carried all the outworks, one after another. There was one, however, that still held out, whose fall would involve that of the whole place. But all my efforts only served at first the better to show me the difficulty, which indeed was something. All this work was perfectly conscious.

Thereupon I left for Mont-Valérien, where I was to go through my military service; so I was very differently occupied. One day, going along the street, the solution of the difficulty which had stopped me suddenly appeared to me. I did not try to go deep into it immediately, and only after my service did I again take up the question...

I shall limit myself to this single example; it is useless to multiply them...

Most striking at first is this appearance of sudden illumination, a manifest sign of long, unconscious prior work. The role of this unconscious work in mathematical invention appears to me incontestable, and traces of it would be found in other cases where it is less evident."

Jacques Hadamard (mathematics)
 Similar experiences have been reported by other mathematicians. One of them is Hadamard:

". . . One phenomenon is certain and I can vouch for its absolute certainty: the sudden and immediate appearance of a solution at the very moment of sudden awakening. On being very abruptly awakened by an external noise, a solution long searched for appeared to me at once without the slightest instant of reflection on my part—the fact was remarkable enough to have struck me unforgettably—and in a quite different direction from any of those which I had previously tried to follow."

Roger Penrose (mathematical physics)
Penrose had been working on what would become known as the event horizons of black holes.

"A colleague... had been visiting from the USA and he was engaging me in voluble conversation on a quite different topic as we walked down the street approaching my office in Birkbeck College in London. The conversation stopped momentarily as we crossed a side road, and resumed again at the other side. Evidently, during those few moments, an idea occurred to me, but then the ensuing conversation blotted it from my mind!

Later in the day, after my colleague had left, I returned to my office. I remember having an odd feeling of elation that I could not account for. I began going through in my mind all the various things that had happened to me during the day, in an attempt to find what it was that had caused this elation. After eliminating numerous inadequate possibilities, I finally brought to mind the thought that I had had while crossing the street—a thought which had momentarily elated me by providing the solution to the problem that had been milling around at the back of my head!"

Andre Marie Ampére (physics)

The unit of electric current is named after Ampére. Here are the circumstances of his first mathematical discovery:

"I gave a shout of joy ... It was seven years ago I proposed to myself a problem which I have not been able to solve directly, but for which I had found by chance a solution, and knew that it was correct, without being able to prove it. The matter often returned to my mind and I had sought twenty times unsuccessfully for this solution. For some days I had carried the idea about with me continually. At last, I do not know how, I found it, together with a large number of curious and new considerations concerning the theory of probability."

Andrew Wiles (mathematics)

Grappling with a solution to Fermat's Last Theorem, Wiles came upon a flaw in his efforts:

A year later, at the point of defeat, he had a revelation.—"It was the most important moment in my working life. Nothing I ever do again will be the same." The very flaw was the key to a strategy he had abandoned years before. In an instant Fermat was proved; a life's ambition achieved; the greatest puzzle of maths was no more.

Karl Friedrich Gauss (mathematics)

Gauss described how he finally proved a theorem on which he had worked unsuccessfully for four years:

"At last two days ago I succeeded, not by dint of painful effort but so to speak by the grace of God. Like a sudden flash of lightning, the enigma was solved. For my part I am unable to name the nature of the thread which connected what I previously knew with that which made my success possible."

Examples: Revelatory Dreams
Niels Bohr (physics)Bohr worked on many different designs trying to figure out what the structure of an atom was, without much success. Finally, one evening he went to sleep. In his dream, he saw the nucleus of the atom, with the electrons spinning around it, just like our solar system with the sun and planets. When he woke up, he knew what his answer was. Using this model, Bohr developed the so-called "old" quantum mechanics, for which he was awarded the Nobel Prize in physics. He spoke often about how it was the dream that gave him the answer he had been searching for.


Albert Einstein (physics)As a young man, Einstein had the following dream:

"I was sledding with my friends at night. I started to slide down the hill but my sled started going faster and faster. I was going so fast that I realized I was approaching the speed of light. I looked up at that point and I saw the stars. They were being refracted into colors I had never seen before. I was filled with a sense of awe. I understood in some way that I was looking at the most important meaning in my life ... my entire scientific career has been a meditation on my dream."

He awoke, soon formulating the constancy of the speed of light and thus, his famous theory of relativity.
 

Otto Loewi (biology)Loewi was working on how nerves transmit impulses. Then one night he fell asleep and had a vivid dream. He dreamed of an experiment that would prove once and for all that transmission of nerve impulses was chemical—not electrical, leading to his discovery of the neurotransmitter acetylcholine.

"The night before Easter Sunday of that year I awoke, turned on the light, and jotted down a few notes on a tiny slip of paper. Then I fell asleep again. It occurred to me at 6 o'clock in the morning that during the night I had written down something most important, but I was unable to decipher the scrawl.

The next night, at 3 o'clock, the idea returned. It was the design of an experiment to determine whether or not the hypothesis of chemical transmission that I had uttered 17 years ago was correct. I got up immediately, went to the laboratory, and performed a single experiment on a frog's heart according to the nocturnal design."

He won the Nobel Prize in medicine for it.


Dmitri Mendeleyev (chemistry)Mendeleyev was trying to make sense of how the chemical elements are organized. One day, he fell asleep while chamber music was being played in the next room. He understood in a dream that the elements were all related to each other in a manner similar to the themes and phrases in music. When he awakened, he was able to write out for the first time the entire periodic table, which formed the basis of modern chemistry.


Srinivasa Ramanujan (mathematics)Ramanujan used to say that a local version of a Hindu deity whispered equations in his ears, or showed him scrolls in his dreams. For instance:

"While asleep I had an unusual experience. There was a red screen ... I was observing it. Suddenly a hand began to write on the screen. I became all attention. That hand wrote a number of results in elliptic integrals. They stuck to my mind. As soon as I woke up, I committed them to writing..."

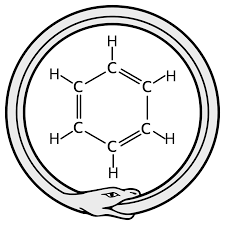Friedrich August von Kekulé (chemistry)

One afternoon, Kekulé fell asleep and dreamt what gave him the clue to a discovery which has been called "the most brilliant piece of prediction to be found in the whole range of organic chemistry"—that the molecules of certain important organic compounds are not linear structures, but closed chains or rings.

"I turned my chair to the fire and dozed. Again the atoms were gambolling before my eyes. This time the smaller groups kept modestly in the background. My mental eye, rendered more acute by repeated visions of this kind, could now distinguish larger structures, of manifold conformation; long rows, sometimes more closely fitted together; all twining and twisting in snakelike motion.

But look! What was that? One of the snakes had seized hold of its own tail, and the form whirled mockingly before my eyes. As if by a flash of lightning I awoke ... Let us learn to dream, gentlemen."

The Stages of Scientific Creativity
Despite persistent research into its nature, the roots of scientific creativity continue to elude inquiring minds. One well-known model maps it as a fourfold process: preparation, incubation, illumination, and verification. (The above examples have focused primarily on the illumination stage.)
What is interesting is that these stages for the physical sciences have their exact counterparts in the spiritual (religious) sciences. These are stages in attaining spiritual maturity, or spiritual perfection.
Preparation, the initial stage, is a period of intense concentration on the problem at hand. This period may last days, months, or even years. This is the period of sowing: "There is nothing for a human being except what s/he has labored for" (53:39). Or, as the Bible puts it, "if a man does not work, neither shall he eat" (2 Thess. 3:10).
The most productive work for scientific creativity is achieved in seclusion and solitude. There is more than a tinge of religious asceticism in the toils of great minds tackling scientific problems. For instance, Andrew Wiles, the mathematician who solved Fermat's Last Theorem, secluded himself in his attic and worked on the problem for almost seven years in near-total secrecy. He shut out everything but Fermat, and confided only in his wife. And then, he had to work another year to correct a flaw in his proof.
In the spiritual sciences, this stage corresponds to active prayer. This is not just a matter of "Lord, give me this and give me that," but conscious labor or "struggle" (mujahada)—more precisely, struggle against the Base Self, opposition to its demands and caprices, doing the opposite of what the Base Self wants. It is also a struggle to wake up. According to Prophetic Sayings: "Human beings are asleep, they wake up when they die," and hence: "Die before you die," so that you may experience spiritual rebirth while still in this world. This too may take years, or even more, for it is no easy matter.
Incubation, the second stage, is where the problem is set aside for a while. All the efforts of rational thought having failed to seize the fortress, as it were, the conscious mind turns to other matters and occupies itself with unrelated things.
This would correspond to detachment in the spiritual sciences: to dissociate oneself from one's efforts, to attain a state of quiet receptivity, of alert relaxation—to abandon the Base Self. As the Sufic saying goes: "You get out from in between, and the Creator at once is seen"—"You" being the Base Self (and its subsequent stages, until the final level is reached).
Illumination occurs when the solution suddenly bubbles up from the unconscious, either fully formed, or in a way that easily leads to success. It can happen either while awake or in a dream. This is the critical stage, yet it would not be possible without the stages that came before it.
What is really interesting about this stage is that it occurs quite without warning, and the scientist involved is at a loss to describe exactly how it was arrived at. "I don't know how I got it" is a frequent exclamation. Apparently, the arts have no monopoly on inspiration: it applies to the sciences as well.
The French mathematician Jacques Hadamard was interested in the psychology of mathematics, and especially in mathematical creativity. He described the experiences of the mathematicians Carl Friedrich Gauss, Henri Poincaré, theoretical physicist Hermann von Helmholtz, and other mathematicians and physicists, as viewing entire solutions with "sudden spontaneousness" (p. 15). In The Emperor's New Mind (1989), mathematical physicist Roger Penrose cites some of Hadamard's cases and adds his own (pp. 541-550). To use his expression, the solutions came to them "in a flash" ("Like a sudden flash of lightning"—Gauss).
In the spiritual sciences, too, this is called illumination—or enlightenment, or "observation" (mushahada). Without struggle, there is no possibility for observation. In the case of prophets, this may be called "revelation" (wahy). For others, it may be called "unveiling" (kashf), where the scales fall from one's eyes and one observes Reality in its full splendor for the first time. (One should, however, guard against premature unveiling—before the ultimate level of selfhood is reached—because it is counterproductive.)
In both kinds of sciences, this is the stage of reception. One is clearly on the receiving end of things: receiving physical or spiritual knowledge from The Source.
Verification, finally, is the stage of consolidation, "rationalization," confirmation by experiment and derivation, where the details and formal proof are worked out painstakingly and systematically. This is basically a stage of convincing one's peers, of making the discovery acceptable to the wider scientific community. By the end of this stage, little may remain that points to the original blinding insight. And yet, without that revelatory moment, no progress would have been possible at all.
And in the spiritual sciences, this is the stage of proclaiming the truth to others, inviting to faith, and also of measuring everything against the yardsticks of the Koran and the Prophetic Way. This is not blind faith, but a faith whose truth has been verified, and can be perceived by all who have eyes to see—just as the truth of a scientific matter in the physical world can be assessed and approved only by scientists competent to pass judgment in that field, and the rest of us have to take them at their word.

Conclusion
Perhaps this sudden illumination is not divine revelation, as that is the province of prophets. But it is certainly inspiration of the purest sort. It is this inspiration that is the original source of knowledge (in both the physical and the spiritual sciences). Working out a laborious proof to "balance the books," to make everything neat and tidy and scientifically respectable, comes later.
As Jacques Barzun commented on Hadamard's study, "it is clear in the end that in any process of creation there lurks a mystery—a mystery at least equal to that of thinking itself." The recipients receive knowledge, but they do not know how they receive it. It comes to them ready-made.
Blaise Pascal was candid enough to state that many of his mathematical insights came directly from God. It is in this context that the full implication of the Master's explanation becomes clear: both spiritual knowledge and physical knowledge are "given," "inspired" ("in-spirited," communicated to the spirit) by God, via His Attribute of Knowledge (ilm).
This also shows that we do not live in a "clockwork universe." God did not create a watch, wind it up, and leave it alone for the rest of eternity. Rather, He actively intervenes in the universe all the time: He is at a task each instant (cf. 55:29). He provides inspiration for all persons—scientists, artists, and mystics—when the appropriate time arrives, and in accordance with their labor. God is beyond the universe, but also within: He is both transcendent and immanent. Moreover, because God is the true Source of scientific knowledge, knowledge/science is the common property of all humanity. So is art, and so is spirituality.



A Note on Sources
The examples of Poincaré, Hadamard, Gauss, Ampére, and Kekulé are taken from Arthur Koestler, The Act of Creation (1964), pp. 115-18. Penrose is taken from Roger Penrose, The Emperor's New Mind (1989), pp. 543-4. Bohr, Einstein, Loewi and Mendeleyev are from Jesse Hong Xiong, The Outline of Parapsychology (2010), pp. 279-80. Wiles is from the BBC. Use was also made of the worlddreambank.org collection (Einstein, Loewi). Ramanujan can be traced back to T. K. Rajagopalan, who is quoted in S. R. Ranganathan, Ramanujan : The Man and the Mathematician (1967), p. 87.